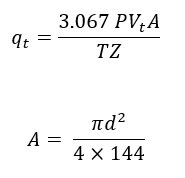Want to read more? Click here for more Well Insights.
UNDERSTANDING CRITICAL FLOWRATE AND LIQUID LOADING
When producing an oil and gas well, liquid loading is a common problem that ultimately leads to a loss in production. Liquid loading occurs when the velocity of the gas fall below the terminal velocity of the liquid droplets. When this occurs, the force of gravity on the liquid droplets is greater than the drag force created by the gas velocity. The result is that the liquid droplets fall to the bottom of the well where they pool up, which ultimately creates hydrostatic pressure against the reservoir.
The diagram above shows that there are two primary forces acting on the liquid droplet, gravity and drag. The drag force is created by the velocity of the gas, and the drag force increases with velocity. In other words, the greater the velocity of the gas, the greater the drag force.
The minimum velocity at which the drag force will overcome the force of gravity is known as the “terminal velocity” or “critical velocity”. The terminal velocity of a liquid droplet can be calculated using the following equation:
Two things to note about the above equation: first is the interfacial tension, which is a parameter that we deal with often. If this is unknown, then the interfacial tension can be assumed to be 20 dynes/cm for oil/condensate, and 60 dynes/cm for water. The second is the Turner correction factor, C. This coefficient was not included in the original work, but after comparing the theoretical equation with actual production data, it was concluded that the theoretical equation needed to be increased by 20% to match actual data. The general rule of thumb is that for surface pressures greater than 500 psia, C = 1.2, and for surface pressure less than 500 psia, C = 1.0.
The above equation calculates the gas velocity needed to prevent liquid loading, however, anyone who has spent more than five minutes in production engineering knows that gas velocity is not a very practical parameter. A more intuitive and well-defined parameter is flowrate. Therefore, once the terminal velocity is known, we can calculate the terminal or “critical” flowrate with the following equations:
The above equation for critical flowrate tells us the minimum flowrate we need to maintain in order to prevent liquid loading. Another way to think of this is that once our flowrate drops below the critical flowrate, we will begin to see liquid loading in our well.
Based on the equations above, here are some important things to note:
As surface pressure increases, so does critical flowrate. This happens because as the pressure increases, the gas takes up less space and thus the velocity decreases. A practical way to think of this is that liquid loading is more likely to occur at higher pressures.
As pipe diameter increases, so does critical flowrate. Smaller tubing diameters will do a better job at lifting water and this will help you hold off liquid loading longer.
As liquid density increases, so does critical flowrate. The heavier the liquid, the stronger the pull of gravity, which means you need more velocity to overcome gravity and lift the droplet.
The graph below shows the relationship between critical flowrate and flowing pressure. Additionally, we see how larger pipe sizes require a much higher flowrate to lift fluid, and illustrates the importance of tubing in terms of deliquefication.
Liquid loading is a common challenge in any producing well. By understanding the physics and using the equations above, we can predict when it will occur, and even design our wells around this in order to give our wells the best opportunity to produce. By using these tools to be proactive about liquid loading, we can see to it that our wells produce as much oil and gas as possible, which ultimately means more money to the bottom line.
Want to read more? Click here for more Well Insights.
You can also subscribe to receive Well Insights updates directly to your inbox.